A life insurance plan for Miss Cathleen
Cynthia helped Miss Cathleen while she was an intern with a life insurance company. Now, Miss Cathleen, aged 48, seeks for financial protection in case she would die around her retirement. To protect her growing-up children she wants to insure a benefit of 40,000 EUR for death between age 55 and 75, as shown below.
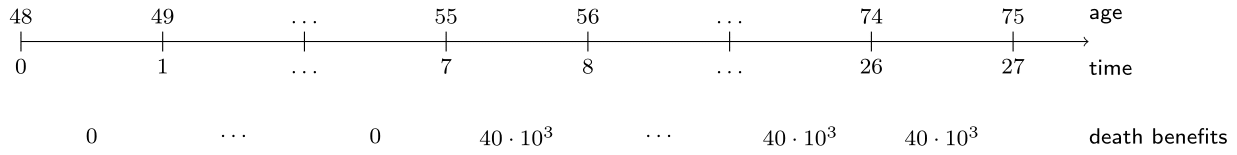
To value this temporary life insurance plan you will once again use the 1999 Belgian female life table, of which the one-year survival probabilities px and mortality rates qx have been preloaded. The assumed interest rate of 5% is available as i.
This exercise is part of the course
Life Insurance Products Valuation in R
Exercise instructions
- Calculate the deferred mortality probabilites \(q_{48}, \: _{1|}q_{48}, \: \ldots, \: _{26|}q_{48}\) of a 48-year-old up to age 75 as the product of multi-year survival probabilities and mortality rates.
- Define the appropriate discount factors at rate
i. - Specify the
benefitsvector as the death benefits of this temporary life insurance. - Compute the expected present value of the plan.
Hands-on interactive exercise
Have a go at this exercise by completing this sample code.
# Deferred mortality probabilites of (48)
kqx <- c(___, ___(px[(___):(___)])) * qx[(___):(___)]
# Discount factors
discount_factors <- (___) ^ - (1:length(kqx))
# Death benefits
benefits <- c(rep(___, ___), rep(___, length(kqx) - 7))
# EPV of the death benefits
EPV_death_benefits <- ___
EPV_death_benefits